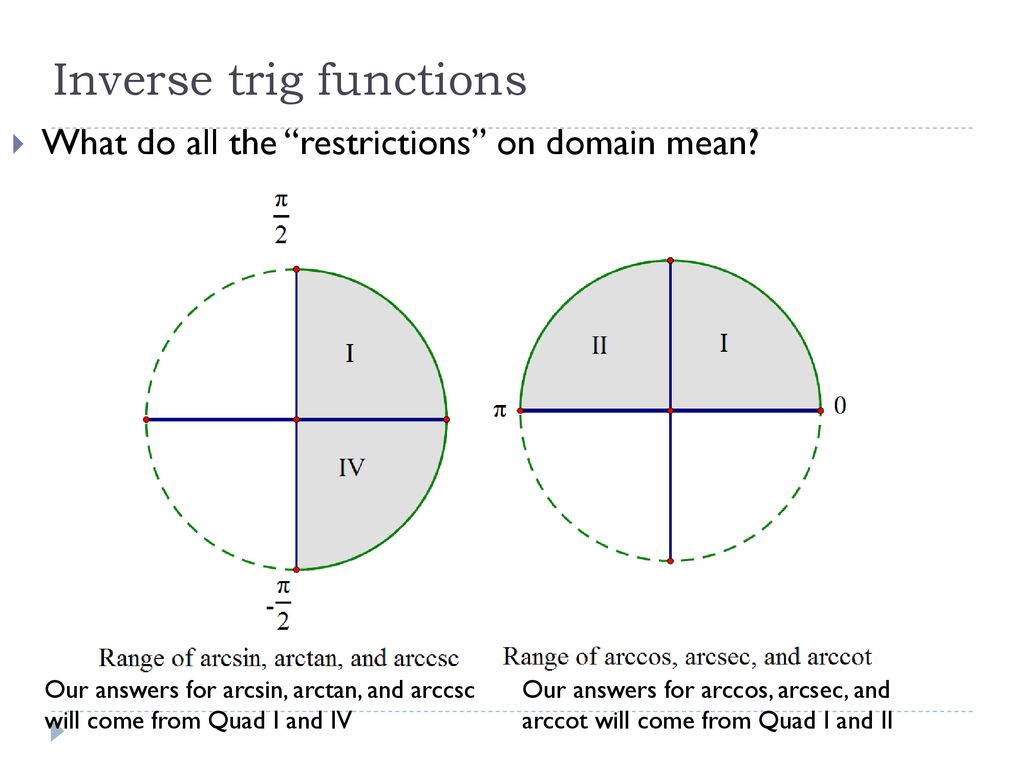
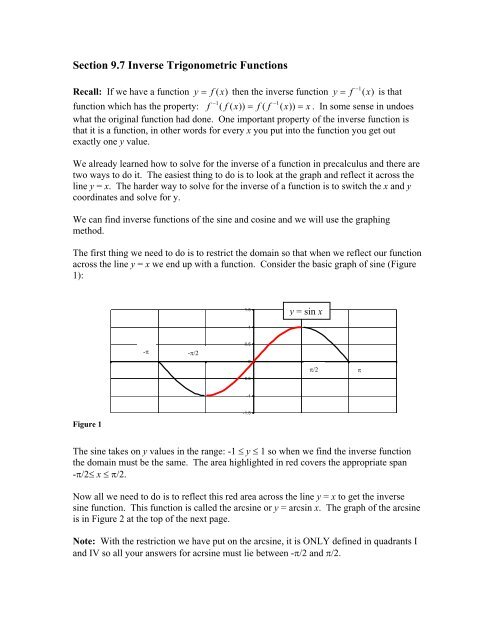
Inverse Trig Functions Domain And Range Restrictions

Bronshtein and semendyayev 1997 p. A different but common convention e g zwillinger 1995 p. In this section you will learn how to find domain and range of inverse trigonometric functions.

domain expert data science domain extensions coming soon domain extensions list 2020 domain extensions for ecommerce domain expert interview questions domain finder calculator online domain extensions other than com domain expert roles and responsibilities

When To Use Restrictions Domain And Range On Trig Functions Mathematics Stack Exchange

Trigonometric Equations Inverse Trigonometric Functions Sparknotes

Inverse Sine Cosine And Tangent Examples Solutions Videos

4 6 Inverse Trig Funtions Christian V S Trig Book Project

Lesson 6 Inverse Trigonometric Functions Latifa S Pre Cal Site

Ppt Lg 2 3 Inverse Trig Functions Powerpoint Presentation Free Download Id 2834780

We need to examine the restrictions on the domain of the original function to determine the inverse.

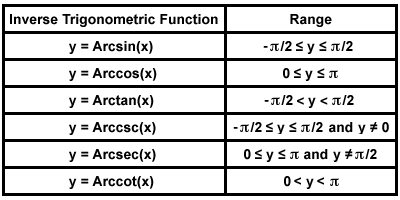
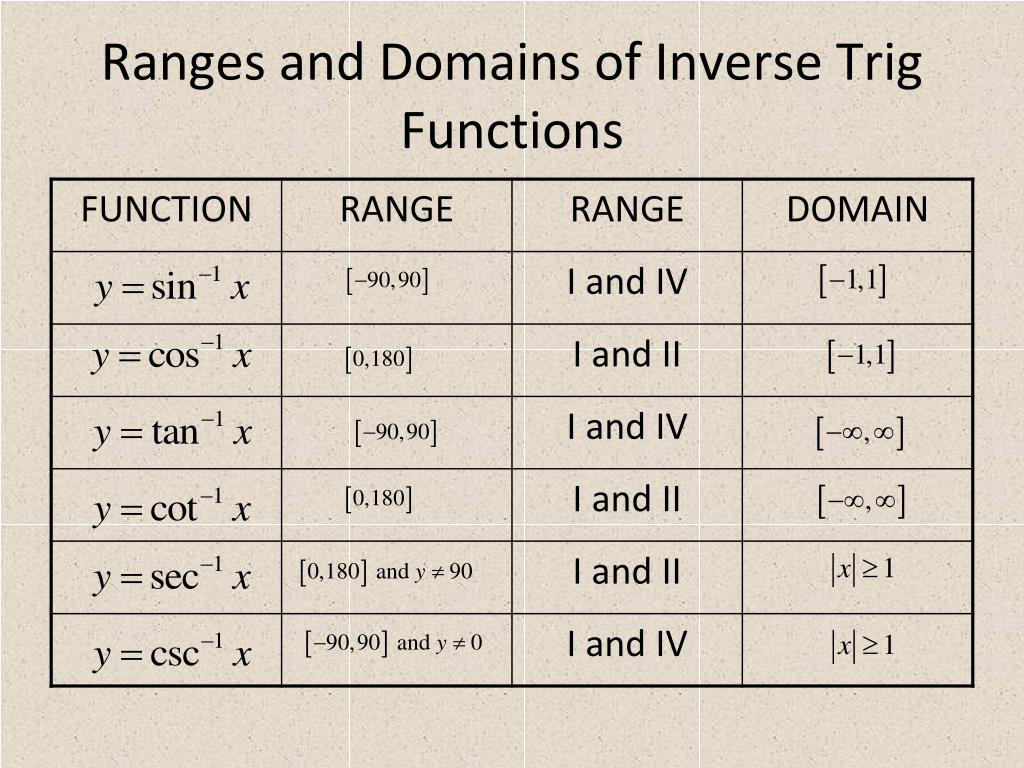
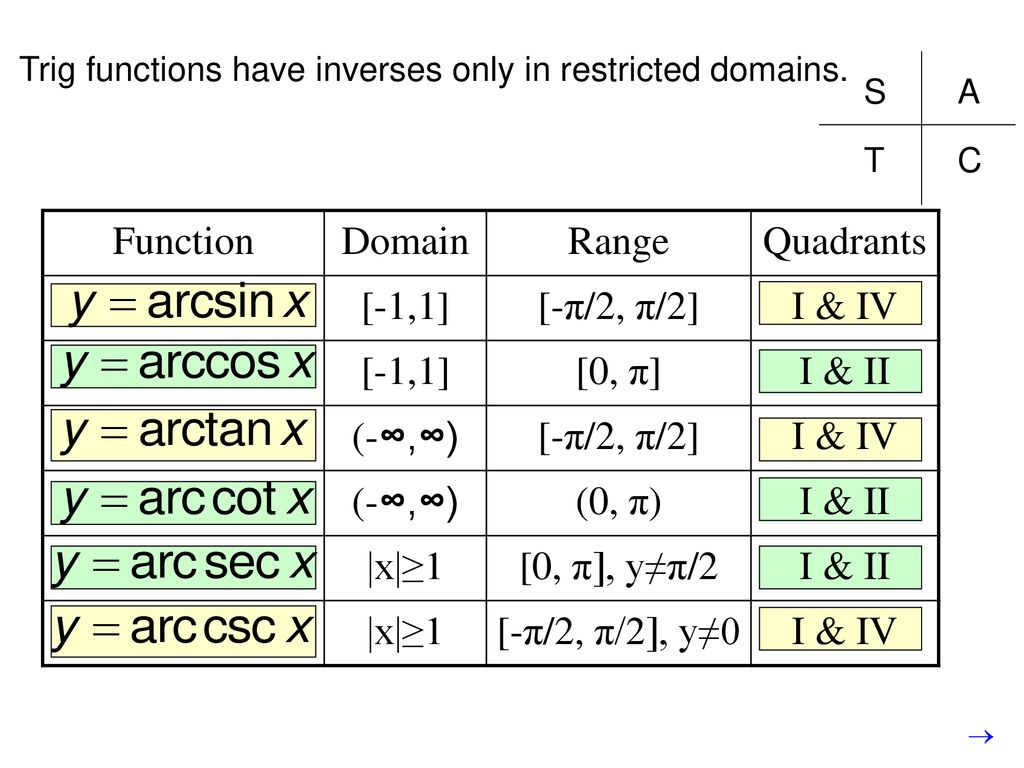
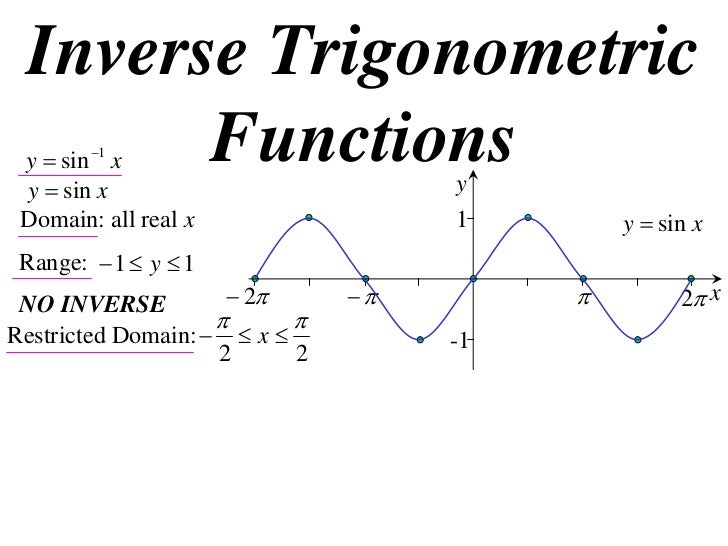
Inverse trig functions domain and range restrictions. We summarize all six. Remember that the number we get when finding the inverse cosine function cos 1 is an angle. Graphically speaking the domain is the portion of the. Extreme care should be taken where examining identities involving inverse trigonometric functions since their range of applicability or precise form may differ.

This question involved the use of the cos 1 button on our calculators. A function that has an inverse has exactly one output belonging to the range for every input belonging to the domain and vice versa to keep inverse trig functions consistent with this definition you have to designate ranges for them that will take care of all the possible input values and not have any duplication. The values x could assume when we reversed the roles of x and y this gave us the values y could assume for this function latex x ge 4 latex so for the inverse we. Since we reversed the roles of x and y for the original f x we looked at the domain.

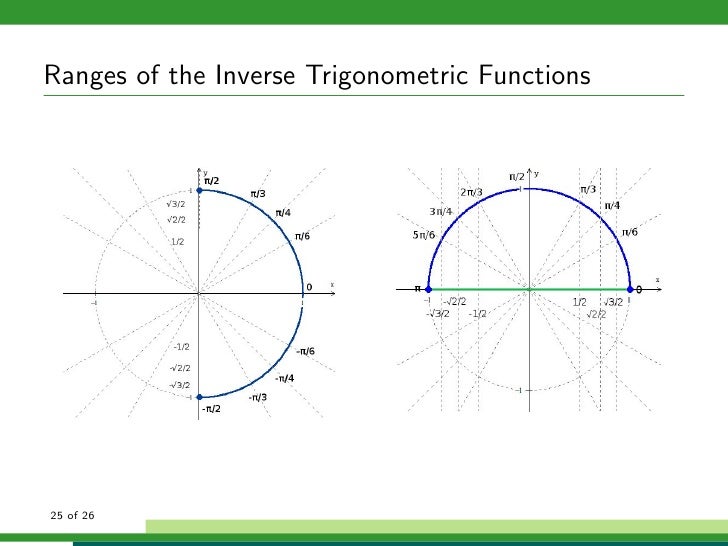
125 defines the range of cot 1 x as 0 pi thus giving a function that is continuous on the real line r. Functions can be determined in a similar fashion. This is relevant for both year 11 and year 12 students preparing for hsc mathematics and hsc maths extension 1 for 2020 and onwards. Their domains must match the ranges of the functions they inverse of course.

Calculations using inverse trig ratios including application of compound angle rules. By mary jane sterling. With a little geometry and reasoning you could have found the entries in the right column if you first derived the entries in the left column. Inverse trigonometric functions domain and range restrictions and their graphs.

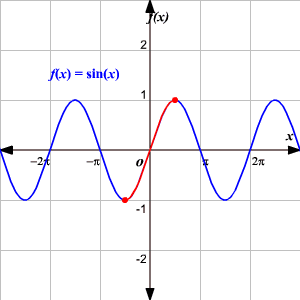
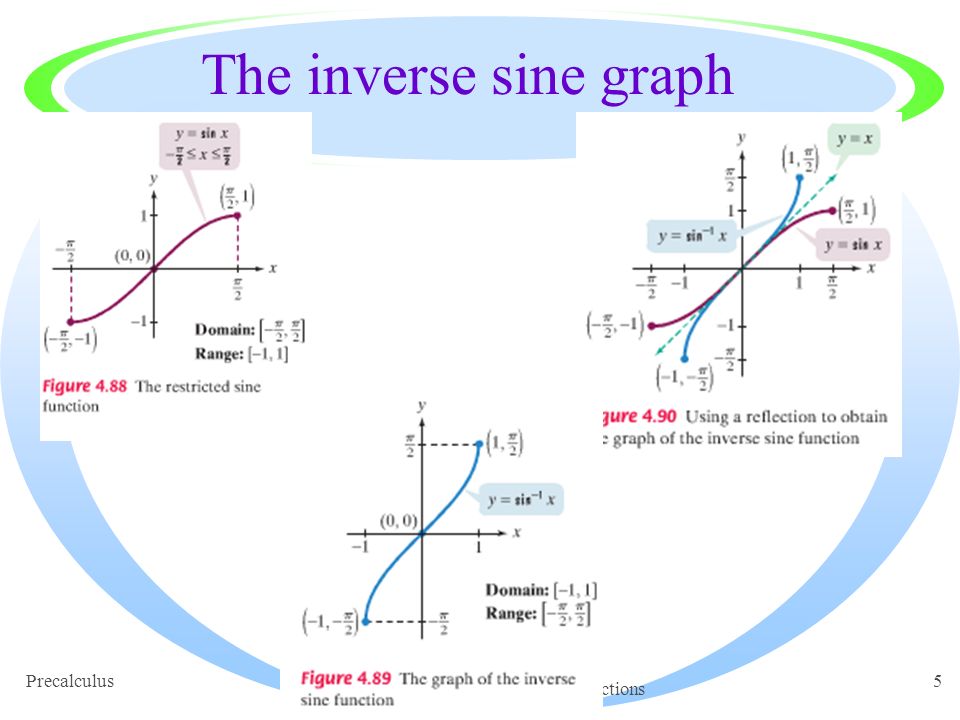
It is actually the ranges that create more of an issue. We found cos 1 0 7 and then considered the quadrants where cosine was positive. To make you to understand the domain and range of an inverse trigonometric function we have given a table which clearly says the domain and range of inverse trigonometric functions. This shows that the sine function is not injective and cannot have an inverse.

This is not a function as written. The range of a function is the list of all possible outputs y values of the function. Domain and range of general functions the domain of a function is the list of all possible inputs x values to the function. We call them that even i do but they are not actually inverse functions.

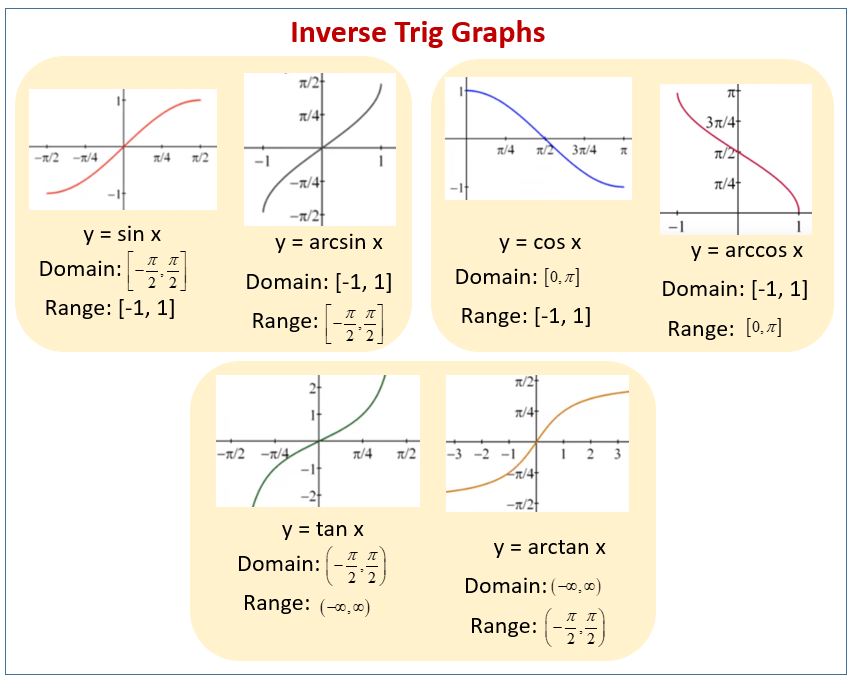
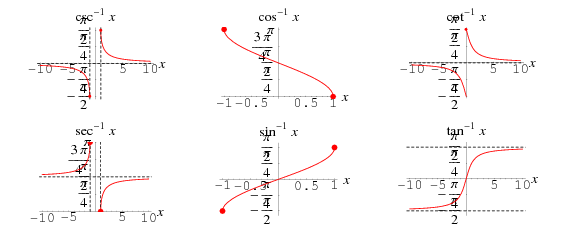
The derivatives of the other four inverse trig. Now we turn our attention to all the inverse trigonometric functions and their graphs.